Duodecimal Numbers
The duodecimal numbers system is a base twelve number system. Its basis, as in the case of the decimal system, is rational.
It is mainly used in Anglo-Saxon societies that use magnitudes of measurement on this basis, such as feet, inches, ounces, etc.
In base twelve, we use the ten digits from 0 to 9, and two variable symbols to represent ten and eleven. There are many situations where A represents ten and B represents eleven, but other symbols are also used.
Examples of Duodecimal Numbers
- 1212 = 1410 (actually, 1᛫121 + 2᛫120)
- 2612 = 3010 (actually, 2᛫121 + 6᛫120)
- 3012 = 3610 = 1006 (actually, 3᛫121 + 0᛫120)
- 5012 = 6010 (actually, 5᛫121 + 0᛫120)
- 6912 = 8110 (actually, 6᛫121 + 9᛫120)
- 7612 = 9010 (actually, 7᛫121 + 6᛫120)
- 8512 = 10110 (actually, 8᛫121 + 5᛫120)
- 10012 = 14410 (actually, 1᛫122 + 0᛫121 + 0᛫120)
- 16012 = 21610 = 10006 (actually, 1᛫122 + 6᛫121 + 0᛫120)
- 1A612 = 27010 (actually, 1᛫122 + 10᛫121 + 6᛫120)
- 26512 = 36510 (actually, 2᛫122 + 6᛫121 + 5᛫120)
- 29412 = 40010 = 10020 (actually, 2᛫122 + 9᛫121 + 4᛫120)
- 40012 = 57610 (actually, 4᛫122 + 0᛫121 + 0᛫120)
- 57612 = 81010 (actually, 5᛫122 + 7᛫121 + 6᛫120)
- 6B412 = 100010 (actually, 6᛫122 + 11᛫121 + 4᛫120)
- 90012 = 129610 = 100006 (actually, 9᛫122 + 0᛫121 + 0᛫120)
- 100012 = 172810 (actually, 1᛫123 + 0᛫122 + 0᛫121 + 0᛫120)
- 11A812 = 200010 (en effet, 1᛫123 + 1᛫122 + 10᛫121 + 8᛫120)
- 245412 = 409610 = 100016 (actually, 2᛫123 + 4᛫122 + 5᛫121 + 4᛫120)
- 396912 = 656110 = 100009 (actually, 3᛫123 + 9᛫122 + 6᛫121 + 9᛫120)
- 460012 = 777610 = 1000006 (en effet, 4᛫123 + 6᛫122 + 0᛫121 + 0᛫120)
- 476812 = 800010 = 100020 (actually, 4᛫123 + 7᛫122 + 6᛫121 + 8᛫120)
- 500012 = 864010 (actually, 5᛫123 + 0᛫122 + 0᛫121 + 0᛫120)
- 789A12 = 1336610 (actually, 7᛫123 + 8᛫122 + 9᛫121 + 10᛫120)
- 1000012 = 2073610 (actually, 1᛫124 + 0᛫123 + 0᛫122 + 0᛫121 + 0᛫120)
- 2300012 = 4665610 = 10000006 (actually, 2᛫124 + 3᛫123 + 0᛫122 + 0᛫121 + 0᛫120)
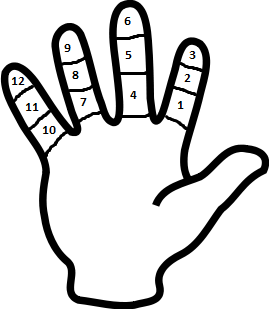
Duodecimal counting with the phalanges. Рыцарь поля, CC0, via Wikimedia Commons
Prime numbers
In any base, a prime number is that integer that cannot be divided by any other integer, except 1 or itself, and yield another integer. As the multiplications are different in the base 12 system than in the base 10 (decimal system), the prime numbers in both bases are different. Thus, in base 12 it turns out that prime numbers can only end in 1, 5, 7 or B (except the numbers 2 and 3).
The number of prime numbers is, as in the other infinite bases (including the decimal), infinite. The first prime numbers in the decimal and duodecimal bases are:
| In duodecimal base | 2 | 3 | 5 | 7 | B | 11 | 15 | 17 | 1B | 25 | 27 | 31 | 35 | 37 | 3B | 45 | 4B | 51 | 57 | 5B | 61 | 67 | 6B | 75 | 81 | 85 | 87 | 8B | 91 | 95 | A7 | AB | B5 | B7 | … |
| Dalam basis desimal | 2 | 3 | 5 | 7 | 11 | 13 | 17 | 19 | 23 | 29 | 31 | 37 | 41 | 43 | 47 | 53 | 59 | 61 | 67 | 71 | 73 | 79 | 83 | 89 | 97 | 101 | 103 | 107 | 109 | 113 | 127 | 131 | 137 | 139 | … |
The multiplication table in the duodecimal or base 12 system is as follows:
| × | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | A | B | 10 |
| 1 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | A | B | 10 |
| 2 | 2 | 4 | 6 | 8 | A | 10 | 12 | 14 | 16 | 18 | 1A | 20 |
| 3 | 3 | 6 | 9 | 10 | 13 | 16 | 19 | 20 | 23 | 26 | 29 | 30 |
| 4 | 4 | 8 | 10 | 14 | 18 | 20 | 24 | 28 | 30 | 34 | 38 | 40 |
| 5 | 5 | A | 13 | 18 | 21 | 26 | 2B | 34 | 39 | 42 | 47 | 50 |
| 6 | 6 | 10 | 16 | 20 | 26 | 30 | 36 | 40 | 46 | 50 | 56 | 60 |
| 7 | 7 | 12 | 19 | 24 | 2B | 36 | 41 | 48 | 53 | 5A | 65 | 70 |
| 8 | 8 | 14 | 20 | 28 | 34 | 40 | 48 | 54 | 60 | 68 | 74 | 80 |
| 9 | 9 | 16 | 23 | 30 | 39 | 46 | 53 | 60 | 69 | 76 | 83 | 90 |
| A | A | 18 | 26 | 34 | 42 | 50 | 5A | 68 | 76 | 84 | 92 | A0 |
| B | B | 1A | 29 | 38 | 47 | 56 | 65 | 74 | 83 | 92 | A1 | B0 |
| 10 | 10 | 20 | 30 | 40 | 50 | 60 | 70 | 80 | 90 | A0 | B0 | 100 |
Examples of arithmetic operations
| Senary (Base 6) | Decimal (Base 10) | Duodecimal (Base 12) | Vigesimal (Base 20) |
|---|---|---|---|
| 140 + 50 = 230 | 60 + 30 = 90 | 50 + 26 = 76 | 30 + 1A = 4A |
| 3430 – 213 = 3213 | 810 – 81 = 729 | 576 – 69 = 509 | 20A – 41 = 1G9 |
| 13132 – 140 = 12552 | 2000 – 60 = 1940 | 11A8 – 50 = 1158 | 500 – 30 = 4H0 |
| 1130 ᛫ 52 = 104000 | 270 ᛫ 32 = 8640 | 1A6 ᛫ 28 = 5000 | DA ᛫ 1C = 11C0 |
| 2400 / 13 = 144 | 576 / 9 = 64 | 400 / 9 = 54 | 18G / 9 = 34 |
| 3430 / 13 = 230 | 810 / 9 = 90 | 576 / 9 = 76 | 20A / 9 = 4A |
| 220 = 30544 | 212 = 4096 | 210 = 2454 | 2C = A4G |
Fractions and irrational numbers
In any rational base positional numeral system (such as decimal and duodecimal), all those irreducible fractions whose denominator contains prime factors other than those that factor the base, will lack finite representation, obtaining for them an infinite series of digits of fractional value (commonly called “decimals”, although it is absurd to use this term for bases other than decimal). Furthermore, this infinite series of digits will present a recurrence period, giving pure recurrence when there is no prime factor in common with the base, and mixed recurrence (the one in which there are fractional digits at the beginning that are not part of the period) when there are at least one prime factor in common with the base.
Examples in duodecimal and Decimal equivalent
| Examples in duodecimal | Decimal equivalent |
|---|---|
| 1 × (5/8) = 0.76 | 1 × (5/8) = 0.625 |
| 100 × (5/8) = 76 | 144 × (5/8) = 90 |
| 576/9 = 76 | 810/9 = 90 |
| 400/9 = 54 | 576/9 = 64 |
| 1A.6 + 7.6 = 26 | 22.5 + 7.5 = 30 |
So, in a duodecimal base, the representation of all those fractions whose denominator contains prime factors other than 2 and 3 is infinite and recursive; while in decimal base this occurs when they are different from 2 and 5:
| Decimal base Prime factors of the base: 2 , 5 | Duodecimal / dozen base Prime factors of the base: 2 , 3 | ||||
| Fraction | Prime factors of the denominator | positional representation | positional representation | Prime factors of the denominator | Fraction |
| 1/2 | two | 0.5 | 0.6 | two | 1/2 |
| 1/3 | 3 | 0, 3 3333333… | 0.4 | 3 | 1/3 |
| 1/4 | two | 0.25 | 0.3 | two | 1/4 |
| 1/5 | 5 | 0.2 | 0, 2497 24972497… | 5 | 1/5 |
| 1/6 | 2 , 3 | 0.1 6 6666666… | 0.2 | 2 , 3 | 1/6 |
| 1/7 | 7 | 0, 142857 142857142857… | 0.186A35 186A35186A35 … | 7 | 1/7 |
| 1/8 | two | 0.125 | 0.16 | two | 1/8 |
| 1/9 | 3 | 0, 1 1111111… | 0.14 | 3 | 1/9 |
| 1/10 | 2 , 5 | 0.1 | 0.1 2497 24972497… | 2 , 5 | 1/Y |
| 11/1 | eleven | 0, 09 09090909… | 0, 1 1111111… | B. | 1 B |
| 1/12 | 2 , 3 | 0.08 3 3333333… | 0.1 | 2 , 3 | 1/10 |
| 1/13 | 13 | 0, 076923 076923076923… | 0.0B 0B0B0B0B … | eleven | 11/1 |
| 1/14 | 2 , 7 | 0.0 714285 714285714285… | 0.0 A35186 A35186A35186… | 2 , 7 | 1/12 |
| 1/15 | 3 , 5 | 0.0 6 6666666… | 0.0 9724 97249724… | 3 , 5 | 1/13 |
| 1/16 | two | 0.0625 | 0.09 | two | 1/14 |
| 1/17 | 17 | 0, 0588235294117647 0588235294117647… | 0, 08579214B36429A7 08579214B36429A7… | fifteen | 1/15 |
| 1/18 | 2 , 3 | 0.0 5 5555555… | 0.08 | 2 , 3 | 1/16 |
| 1/19 | 19 | 0, 052631578947368421 052631578947368421… | 0.076B45 076B45076B45 … | 17 | 1/17 |
| 1/20 | 2 , 5 | 0.05 | 0.0 7249 72497249… | 2 , 5 | 1/18 |
| 1/21 | 3 , 7 | 0, 047619 047619047619… | 0.0 6A3518 6A35186A3518… | 3 , 7 | 1/19 |
| 1/22 | 2 , 11 | 0.0 45 45454545… | 0.0 6 6666666… | 2 , B | 1/1A |
| 1/23 | 23 | 0, 0434782608695652173913 043478260869565… | 0, 06316948421 06316948421… | 1 B | 1/1B |
| 1/24 | 2 , 3 | 0.041 6 6666666… | 0.06 | 2 , 3 | 1/20 |
| 1/25 | 5 | 0.04 | 0, 05915343A0B6 05915343A0B6… | 5 | 1/21 |
| 1/26 | 2 , 13 | 0.0 384615 384615384615… | 0.0 56 565656565… | 2 , 11 | 1/22 |
| 1/27 | 3 | 0, 037 037037037… | 0.054 | 3 | 1/23 |
| 1/28 | 2 , 7 | 0.03 571428 571428571428… | 0.0 5186A3 5186A35186A3… | 2 , 7 | 1/24 |
| 1/29 | 29 | 0, 0344827586206896551724137931 0344827586… | 0.04B7 04B704B7 … | 25 | 1/25 |
| 1/30 | 2 , 3 , 5 | 0.0 3 3333333… | 0.0 4972 49724972… | 2 , 3 , 5 | 1/26 |
| 1/31 | 31 | 0, 032258064516129 032258064516129… | 0.0478AA093598166B74311B28623A55 0478AA … | 27 | 1/27 |
| 1/32 | two | 0.03125 | 0.046 | two | 1/28 |
| 1/33 | 3 , 11 | 0, 03 03030303… | 0.0 4 4444444… | 3 , B | 1/29 |
| 1/34 | 2 , 17 | 0.0 2941176470588235 2941176470588235… | 0.0 429A708579214B36 429A708579214B36… | 2 , 15 | 1/2A |
| 1/35 | 5 , 7 | 0.0 285714 285714285714… | 0.0414559B3931 0414559B3931 … | 5 , 7 | 1/2B |
| 1/36 | 2 , 3 | 0.02 7 7777777… | 0.04 | 2 , 3 | 1/30 |
On the other hand, in any positional numeral system with a rational base, every irrational number not only lacks a finite representation, but also its infinite series of digits lacks a recurrence period. The first few digits of the base duodecimal representation of several of the most important irrational numbers are given below:
| irrational number | in decimal base | in duodecimal base |
| π (pi, the ratio of circumference to diameter ) | 3.141592653589793238462643… (~ 3.1416) | 3.184809493B918664573A6211… (~ 3.1848) |
| e (the base of the natural or natural logarithm ) | 2.718281828459… (~ 2.718) | 2.875236069821… (~ 2.875) |
| φ (fi, the golden number or golden ratio ) | 1.618033988749… (~ 1.618) | 1.74BB67728022… (~1.75) |
| √2 (the length of the diagonal of a unit square ) | 1.414213562373… (~ 1.414) | 1.4B79170A07B7…(~1.4B8) |
| √3 (the length of the diagonal of a unit cube , or twice the height of an equilateral triangle ) | 1.732050807568… (~ 1.732) | 1,894B97BB967B… (~ 1,895) |
| √5 (the length of the diagonal of a 1×2 rectangle ) | 2,236067977499… (~ 2,236) | 2.29BB13254051…(~2.2A) |
Irrational numbers
The representations of irrational numbers in any positional number system (including decimal and duodecimal) neither terminate nor repeat. The following table gives the first digits for some important algebraic and transcendental numbers in both decimal and duodecimal.
| Algebraic irrational number | In decimal | In duodecimal |
|---|---|---|
| √2, the square root of 2 | 1.414213562373… | 1;4B79170A07B8… |
| φ (phi), the golden ratio = {\displaystyle {\tfrac {1+{\sqrt {5}}}{2}}} | 1.618033988749… | 1;74BB6772802A… |
| Transcendental number | In decimal | In duodecimal |
| π (pi), the ratio of a circle’s circumference to its diameter | 3.141592653589… | 3;184809493B91… |
| e, the base of the natural logarithm | 2.718281828459… | 2;875236069821… |
The first digits in duodecimal base of another notable number, the Euler-Mascheroni constant , but for which at the moment it is unknown if it is rational or irrational:
| Number | in decimal base | in duodecimal base |
| γ (the limiting difference between the harmonic series and the natural logarithm) | 0.577215664901… (~ 0.577) | 0.6B15188A6758… (~0.7) |
Sources: PinterPandai, MathIsFun



