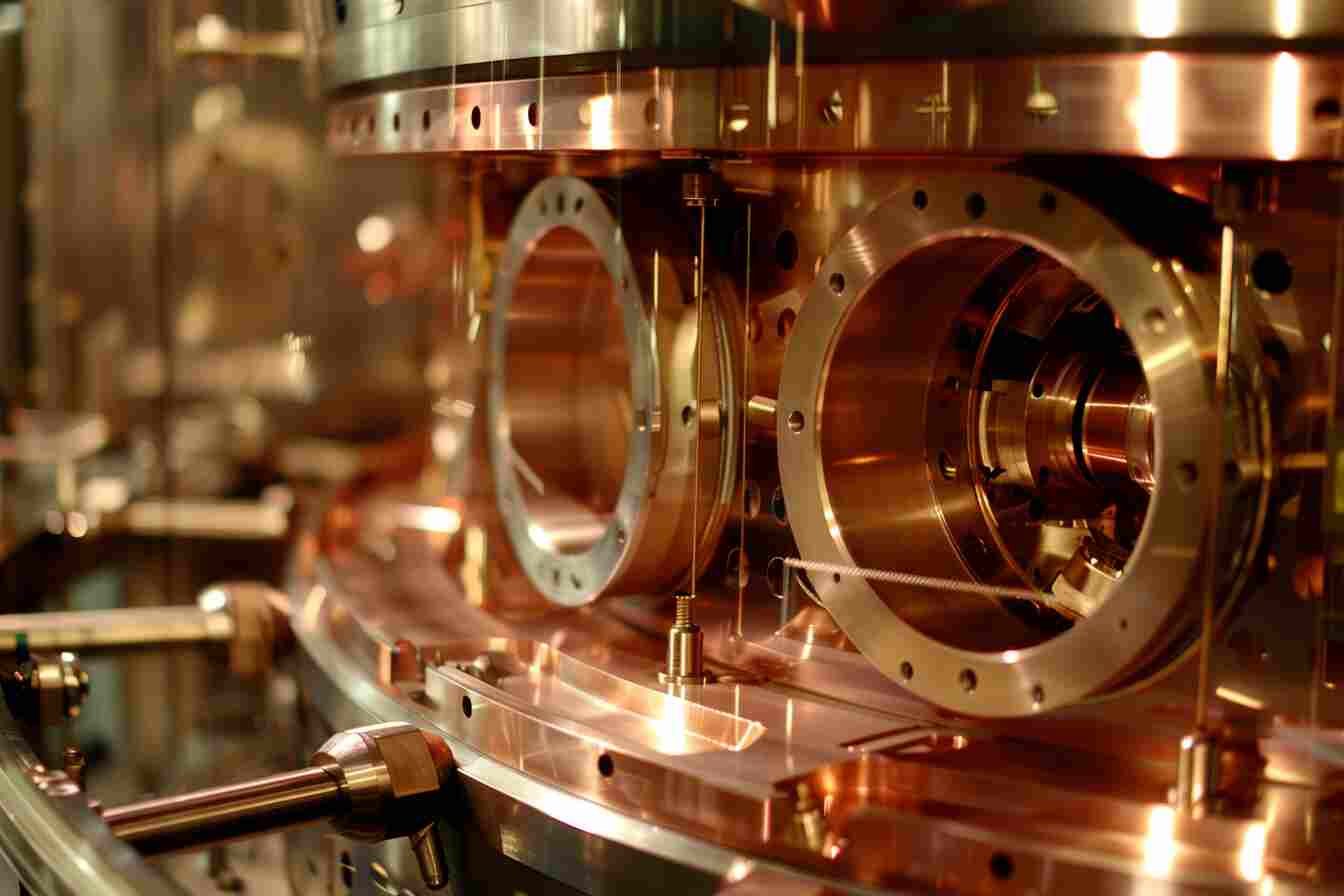
Cyclotron, Synchrocyclotron and Synchrotron (Physics)
Particle accelerator physics is widely used in current research. The term “cyclotron” sounds straight out of a science fiction movie. In fact, cyclotrons are particle accelerators, devices that propel charged particles at very high speeds using electromagnetic fields. They are used to produce radioisotopes that are used to make “radiopharmaceuticals,” drugs that can diagnose and treat cancer.
In particular, such accelerators called synchrotrons allow the use of particles such as electrons or positrons to generate synchrotron radiation which can then be used to study matter at unprecedented scales. (Note that another type of synchrotron distinguished by its construction constraints makes it possible to study the strong interaction by accelerating protons or antiprotons).
Consequently, this type of instrument is developed in many countries of the world in order to serve different fields of research such as the study of materials, medicine, biology or even archeology. Thus, synchrotron technology is now very accessible, this opens the door to this cutting-edge technology to many specialist researchers in various disciplines around the world (studies of proteins allowing photosynthesis, drug development, development of new materials, etc.).
The cyclotron (non-relativistic particles)
The cyclotron is a type of particle accelerator invented by Ernest Orlando Lawrence and Milton Stanley Livingston of the University of California, Berkeley in the early 1930s.

Cyclotron 70 MeV installed at the University of Nantes (France) in 2008. The cyclotron is designed for accelarating protons, deutons and alpha particles. Popocatomar, CC BY-SA 4.0, via Wikimedia Commons
Description of the device
The cyclotron consists of two “Dees hollow semicircular electrodes” separated by a small space. the whole is immersed in a field magnetic B constant. Between the two Dees, we have a sinusoidal voltage U ( U = Um cos(qB/m t + φ)) and therefore a sinusoidal electric field E (E = Em cos(qB/m t + φ)). We choose φ so that U is maximum when the particle passes between the “Dees”.
The particle is accelerated between the Dees and describes a semicircle of radius R inside the Dees. She is
therefore accelerated twice per turn.
Inside the Dees, the particle of mass m and load q undergoes the Lorentz force F = q v X B
Between the Dees, the particle is accelerated by the force electric F = q E
Particle trajectory
v being perpendicular to B, we have F = q v B
F being perpendicular to v, the tangential acceleration at = dv/dt is zero, therefore the modulus of v is constant and therefore the momentum p = γ m v is constant.
When the particle rotates by an angle dθ the vector p also rotates by dθ so its variation dp
is p dθ
According to Newton’s second law: dp/dt = F = q v B
p dθ/dt = p ω = p v/R = q v B
p/R = qB
R = p /(q B) = γ m v /(qB) = constant
Between the Dees, the particle is accelerated so ΔEc
= q Um. At each turn the particle has been accelerated twice so ΔE
c = 2 q Um
After n revolutions, Ec = 2 n q Um (The initial speed is negligible)
The cyclotron is used to accelerate heavy particles (protons, ions, etc.) to non-relativistic speeds (v << c and γ = 1).
R = m v /(qB)
Ec = 1/2 m v² = 2 n q Um so v = (4 n q Um/m)½
R = m (4 n q Um/m)½/(qB) = (4 n m Um/(qB²))½
R = (4 n m Um/(qB²))½
v = q/m BR
n = qB²R²/(4 m Um) or R = (4 mUm/(qB²) n)½ (R is proportional to n½)
Example: proton ( m = 1.67.10-27 kg and q = 1.6.10-19 C ) Rmax = 0.3 m, B = 0.8 T,
U = 5000V
vmax = 2.3.107 m/s
nmax = 276
Cyclotron period
T = 2π R/v
T = 2π m v /(qB)/v = 2π m/(qB) = constant
T = 2π m/(qB)
ω = 2π/T = qB/m It is therefore necessary to apply a pulsation voltage ω = qB/m or of frequency f = qB/(2π m)
Example: proton ( m = 1.67.10-27 kg and q = 1.6.10-19 C ) R = 0.3 m, B = 0.8 T, U = 5000 V, f = 12.2 MHz
R = mv /(qB)
Ec = 1/2 m v² = 2 n q Um jadi v = (4 n q Um/m)½
R = m (4 n q Um/m)½/(qB) = (4 n m Um/(qB²))½
R = (4 n m Um/(qB²))½
v = q/m BR
n = qB²R²/(4 m Um) atau R = (4 mUm/(qB²) n)½ (R sebanding dengan n½)
Contoh : proton ( m = 1.67.10-27 kg dan q = 1.6.10-19 C ) Rmax = 0.3 m, B = 0.8 T,
U = 5000V
vmaks = 2.3.107 m/s
nmaks = 276
= periode siklotron
T = 2π R/v
T = 2π m v /(qB)/v = 2π m/(qB) = konstan
T = 2π m/(qB)
= 2π/T = qB/m Oleh karena itu diperlukan tegangan pulsasi = qB/m atau frekuensi f = qB/(2π m)
Contoh : proton ( m = 1.67.10-27 kg dan q = 1.6.10-19 C ) R = 0.3 m, B = 0.8 T, U = 5000 V, f = 12,2 MHz
R = mv /(qB)
Ec = 1/2 m v² = 2 n q Um so v = (4 n q Um/m)½
R = m (4 n q Um/m)½/(qB) = (4 n m Um/(qB²))½
R = (4 n m Um/(qB²))½
v = q/m BR
n = qB²R²/(4 mUm) or R = (4 mUm/(qB²) n)½ (R is proportional to n½)
Example: proton ( m = 1.67.10-27 kg and q = 1.6.10-19 C ) Rmax = 0.3 m, B = 0.8 T,
U = 5000V
vmax = 2.3.107 m/s
nmax = 276
= cyclotron period
T = 2π R/v
T = 2π m v /(qB)/v = 2π m/(qB) = constant
T = 2π m/(qB)
= 2π/T = qB/m Therefore, we need pulsating voltage = qB/m or frequency f = qB/(2π m)
Example: proton ( m = 1.67.10-27 kg and q = 1.6.10-19 C ) R = 0.3 m, B = 0.8 T, U = 5000 V, f = 12.2 MHz
Description of the device The device is identical to the cyclotron. The only difference is that the frequency of the voltage is not constant, it is synchronized with that of the particles. Hence its name synchrocyclotron.
Synchrocyclotron (relativistic particle)
A synchrocyclotron is a cyclotron in which the frequency of the electric field is changed (gradually decreased) to compensate for the mass gain of the accelerated particles as their speed begins to approach the speed of light.
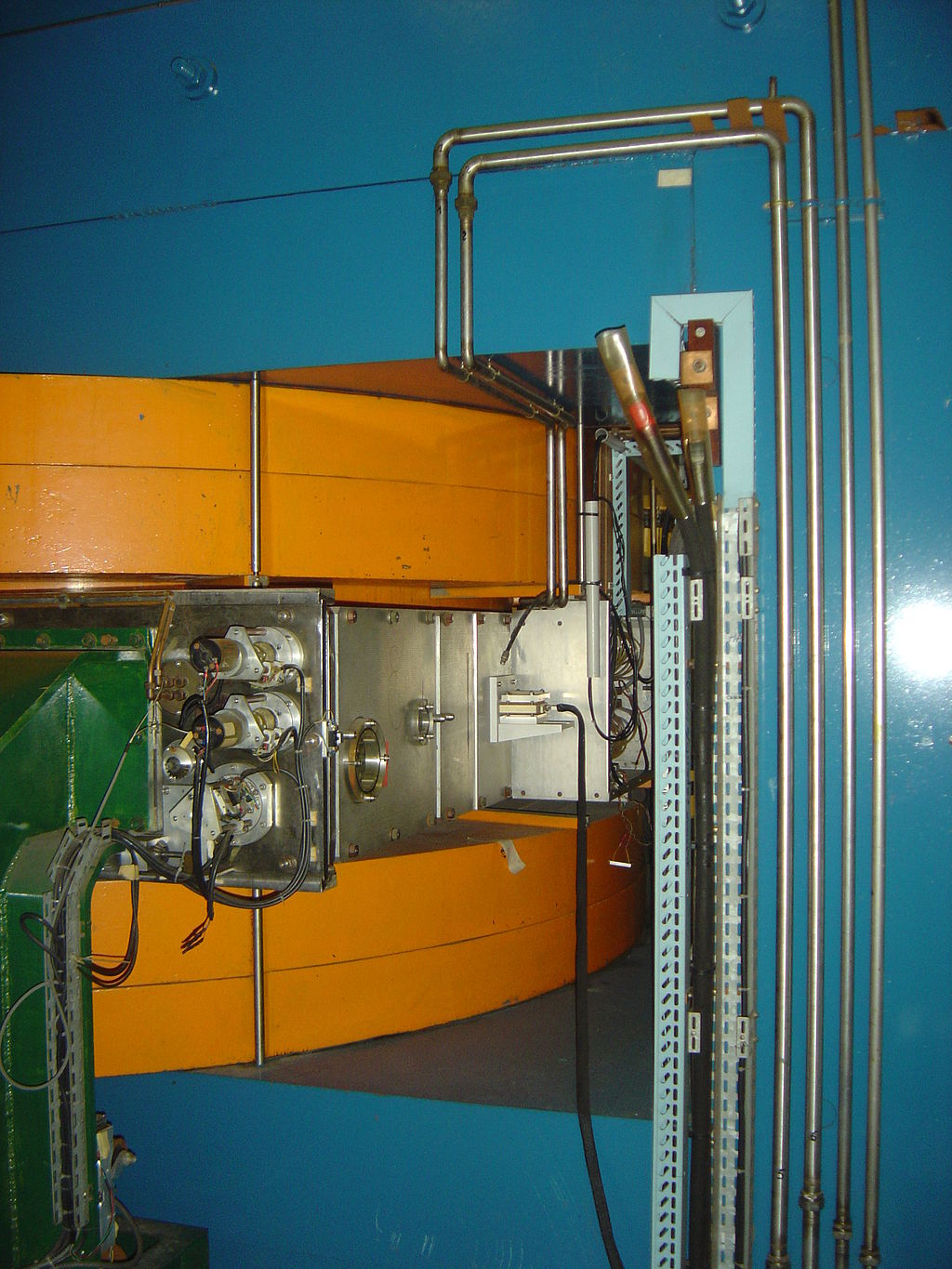
A part of the Orsay synchrocyclotron magnet, now used by the proton therapy centre. David Monniaux, CC BY-SA 3.0, via Wikimedia Commons
Device description
This device is identical to the cyclotron. The only difference is that the frequency of the voltage is not constant, it is synchronized with the particles. Hence the name synchrocyclotron.
Particle trajectory
v being perpendicular to B, we have F = q v B
F being perpendicular to v, the tangential acceleration at = dv/dt is zero, therefore the modulus of v is constant and therefore the momentum p = γ m v is constant.
When the particle rotates by an angle dθ the vector p also rotates by dθ so its variation dp is equal to p dθ
According to Newton’s second law: dp/dt = F = q v B
p dθ/dt = p ω = p v/R = q v B
p/R = qB
R = p /(q B) = γ m v /(qB) = constant
Between the Dees, the particle is accelerated so ΔEc
= q Um. At each turn the particle was accelerated twice so ΔEc = 2 q Um
After n revolutions, Ec = 2 n q Um (The initial speed is negligible)
R = γ m v /(qB)
Ec = (γ – 1) mc² = 2 n q Um
After some calculations, we deduce:
R = mc/(qB) ((1 + 2 n q Um/(mc²))² -1)½
γ = (1 + (qBR/(mc))²)½
v = c ((qBR/(mc))²/(1 + (qBR/(mc))²))½
n = ((1 + (qBR/(mc))²)½ -1) mc²/(2qUm)
If the particle is ultrarelativistic with negligible radiation loss (qBR >> mc)
γ = qBR/(mc)
v=c
n = cBR/(2Um) or R = 2Um/(cB) n (R is proportional to n and is independent of the nature of the particle!)
Example: electron ( m = 0.91. 10-27 kg and q = 1.6.10-19 C ) Rmax
= 0.3m,
B = 0.1 T, Um
= 5000V
γmax = 18
nmax = 900
Synchrocyclotron period
T = 2π R/v = 2π γ m/(qB)
γ = (1 + (qBR/(mc))²)½
T = 2π m/(qB) (1 + (qBR/(mc))²)½
ω = 2π/T = qB/(m(1 + (qBR/(mc))²)½) It is therefore necessary to apply a variable frequency voltage f = qB/(2π m (1 + (qBR/(mc))) ²)½)
If the particle is ultrarelativistic (qBR >> mc)
T = 2 π R/c ( Which is normal since then v = c )
f = 2π/T = c/R
The synchrotron (ultrarelativistic electrons)
Is a type of compact particle accelerator which produces radioactive isotopes, producing the imaging isotopes used for the PET-CT scanner.
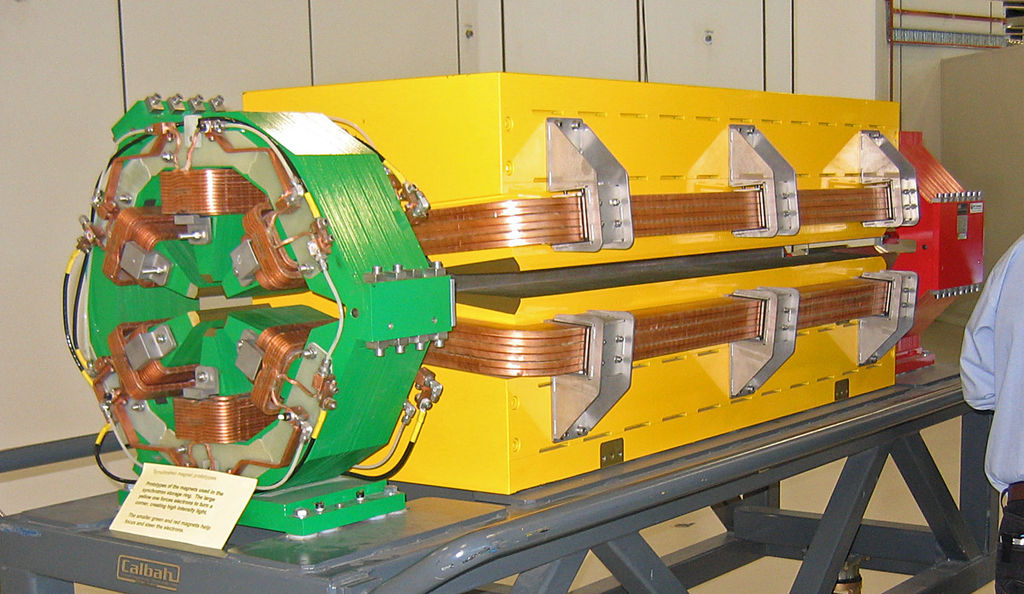
Sextupole magnets used for focussing the electron beam in the storage ring, as used at the Australian Synchrotron, Clayton, Victoria. The larger yellow one is a dipole bending magnet, used to bend the electron beam and produce the synchrotron light. The green one is a sextupole magnet, the red one (at back) a quadrupole magnet – these are used mainly to focus the electron beam. jjron, CC BY-SA 3.0, via Wikimedia Commons
Description of the device
The principle is the same as for the synchrocyclotron except that the trajectory is maintained fixed by adapting the magnetic field B which increases as the momentum increases and particles are injected into it which have already previously been accelerated to ultrarelativistic speeds.
The synchrotron is notably used to produce powerful electromagnetic radiation.
Particle trajectory
v ~ c so γ >> 1
R = γ m c /(qB)
B = γ m c/(qR)
On its trajectory, the particle is accelerated k times so after one turn, ΔEc = k q Um – Elum (Elum being the energy lost by radiation.)
B = Ec/(qcR)
Example: electron ( m = 0.91.10-27 kg and q = 1.6. 10-19 C ) R = 0.5 m
B = 0.3375T
Light emission by an accelerated charged particle
An accelerated charged particle emits powerful electromagnetic radiation
P
P = γ4 q²/(6πε0c3)ap² (ap acceleration perpendicular to the direction of emission)
This power is important only if γ is very large (ultrarelativistic motion v = c). The emission then takes place essentially in a cone with an angle at the top close to 1/γ which is therefore very small. The wavelength is then close to λ = πR/γ²
Under these conditions, the loss of energy due to the electromagnetic emission causes the kinetic energy of the particle to drop, which requires supplying it with energy to maintain its speed. This energy can become completely prohibitive when one wants to obtain very large values of γ. To minimize it, it is necessary to decrease to
p by taking a large value for the radius R. The LHC is 27 km in circumference…
Example: electron in the LHC with energy E = 100 GeV.
We obtain γ = 2. 105, ap = c²/R and P = 3.6.10-6 W from λ = 3.4. 10–7 m (UV)
If we want to maintain the energy of 1012 electrons, which is not huge, we will need a power of 3.6 MW!
For a proton with a hundred times greater energy E = 10000 GeV. We obtain
γ = 10000, ap = c²/R and P = 2.5. 10–11 W, which is very reasonable.
Sources: PinterPandai, CERN, Fedoruk Centre, Physics Tasks
Main photo powered by Midjourney